4.1 PN 접합(PN Junction)

4.1.0 PN 접합의 기본 이론  

 > PN 접합은 P type 반도체와 N type 반도체를 붙인 것이다. 

 

 > P, N type 반도체 간의 캐리어 농도차이로 인해 접합 후 확산이 이루어지게 된다. 

 

 > 접합부분에 전자와 정공이 Recombination되어 캐리어가 거의 없는 공핍층(Depletion Layer)가 만들어진다. 

 

 > 공핍층에는 이온들의 전하 차이로인해 내부 전압이 생기게되고 PN 방향의 반대로 형성된다. 

 

 > PN 방향(정방향)으로 내부 전압보다 높은 전압이 가해지면 전류가 흐른다. 

 

 > 반대로 NP(반대방향)으로 전압이 가해지면 공핍층이 커지고 내부 전압이 커지면서 전류가 흐르지 못하게 된다. 

 

 > PN 접합은 전류가  PN 방향으로만 흐르는 정류 특성을 가지며 이러한 소자를 다이오드(Diode)라고 한다. 

 

 > PN 접합은 모든 형태의 트랜지스터(Transistor)에 존재하고 기본이되는 개념이기 때문에 중요한 내용이다.

 

 

4.1.1 PN 접합의 에너지 밴드 다이어그램 

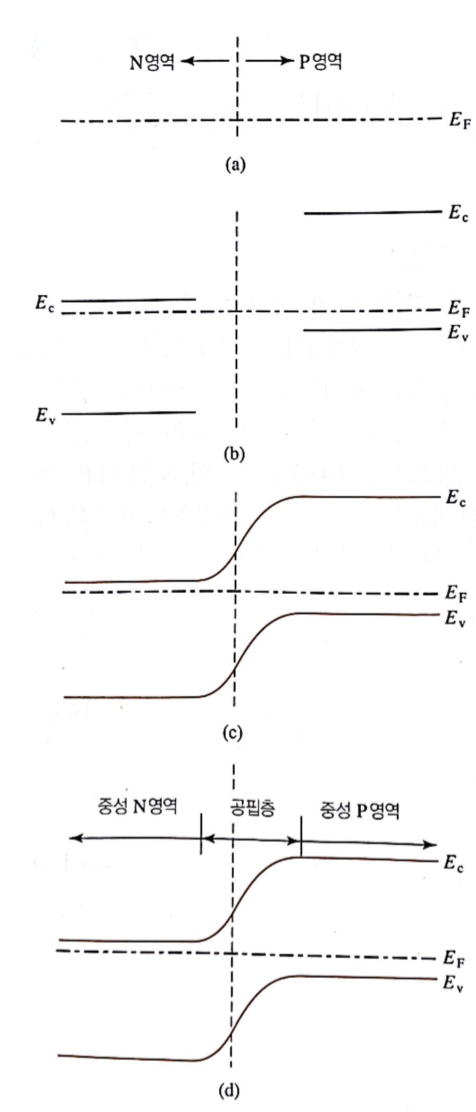
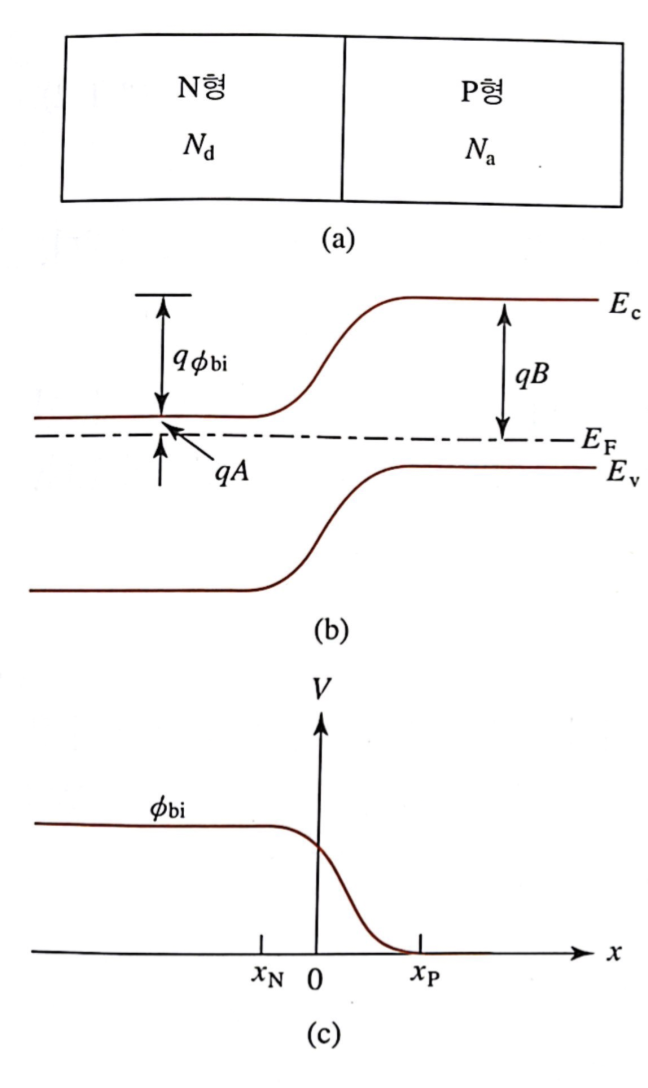
 > 평형상태에서는 단 하나의 페르미 레벨(Ef)을 갖는다. 

 

 > Ef는 N, P type 반도체에 일정한 선을 갖는다. 

 

 > N type의 Ec(Conduction Band)는 Ef와 가깝고 P type의 Ev(Valence Band)는 Ef와 가깝다. 

 

 > 하나의 페르미 레벨 기준으로 N, P type의 EBD(Energy Band Diagram)을 연결시키면 그림 1과 같은 부드러운 곡선이 그련진다. 

 

 > N, P층 사이에 공핍층으로 나누어지고 공핍층의 Ef는 Ev와 Ec에 가깝지 않다. 

 

 > 그러므로 공핍층에는 전자와 정공 농도가 매우 낮다.

그림 1. PN Junction EBD

 

4.1.2 내부 전위 

 > 그림 2 (b)를 보면 Ec와  Ev가 평평하지 않고 굴곡이져있다. 

 

 > 이는 전압 차이가 있는것을 나타내고 내부 전압(Built in Voltage)라고 한다. 

 

 > 경계면을 이루는 경우에는 언제나 존재하고 Na(Accepter 농도), Nd(Donor 농도)에 의해 결정된다.  

 

그림 2. Built In Voltage

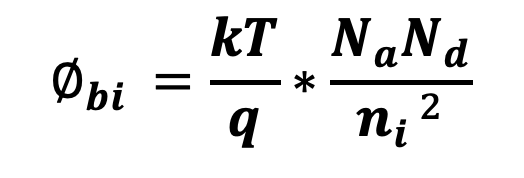
4.1.3 포아송 방정식 

 > 거리를 시간으로 미분하면 속도가 되고, 속도를 시간으로 미분하면 가속도를 구하는 것처럼 전압도 동일한다. 

 

 > 전압을 미분하면 전계가되고, 전계를 한번 더 미분하면 전하량이 된다. 

 

 > 이를 포아송 방정식(Poisson's Equation)이라고 한다. 

 

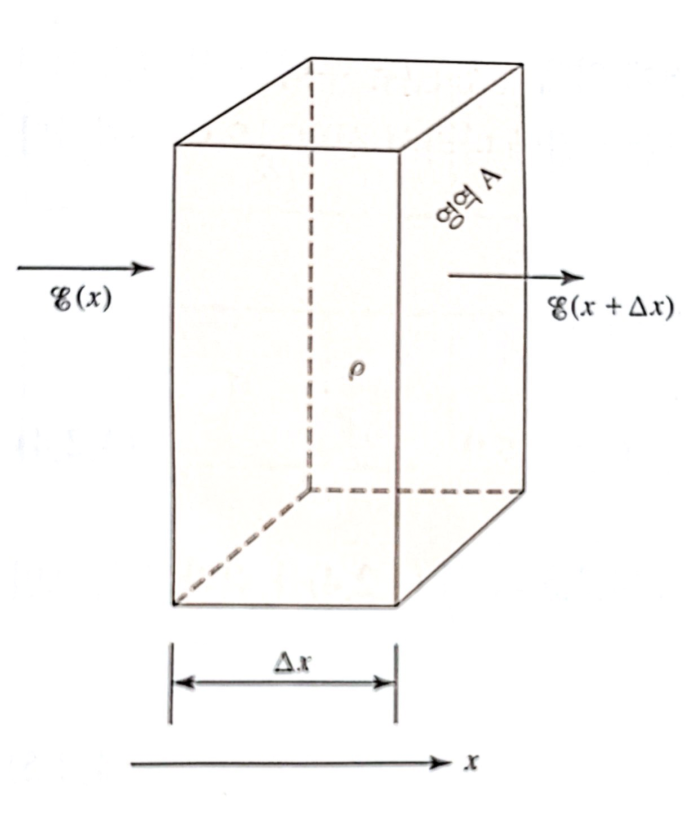
 > 구하는 방법은 단위 체적에 들어가는 전계와 나가는 전계의 차이를 전하량으로 구할 수 있다. 

 

 > 포아송 방정식은 전하량 밀도가 주어질 때 전위 분포를 찾는데 유용한 식이다. 

 

 

그림 3. Poisson's Equation

 

 

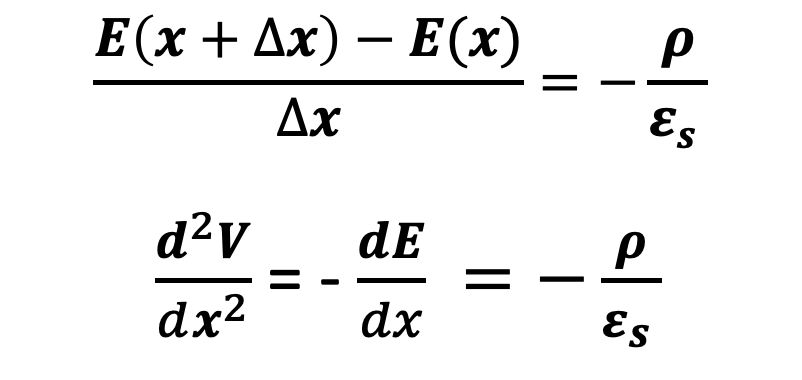
4.1.0 Basic Theory of PN Junction

 

•A PN junction is formed by joining P-type and N-type semiconductors.

 

•Diffusion occurs after the junction due to the difference in carrier concentration between P and N-type semiconductors.

 

•Electrons and holes recombine at the junction, creating a depletion layer with few carriers.

 

•The depletion layer generates an internal voltage due to the charge difference between ions and forms in the direction of PN.

 

•When a higher voltage is applied in the PN direction (forward bias), current flows.

 

•Conversely, when voltage is applied in the NP direction (reverse bias), the depletion layer widens, and the increasing internal voltage prevents current flow.

 

•PN junction exhibits the rectifying characteristic of current flowing in the PN direction only, and such a component is called a diode.

 

•PN junctions exist in all forms of transistors and are a fundamental concept, making them important.

 

 

4.1.1 Energy Band Diagram of PN Junction

 

•In the equilibrium state, it has only one Fermi level (Ef).

 

•Ef maintains a constant level in N and P-type semiconductors.

 

•The Conduction Band (Ec) of N-type is close to Ef, and the Valence Band (Ev) of P-type is close to Ef.

 

•Connecting the EBD (Energy Band Diagram) of N and P-type with a single Fermi level results in a smooth curve as in Figure 1.

 

•A depletion layer is formed between the N and P layers, and the Ef in the depletion layer is not close to Ev and Ec.

 

•Therefore, the electron and hole concentrations in the depletion layer are very low.

 

Figure 1. PN Junction EBD

 

 

4.1.2 Built-In Voltage

•Figure 2 (b) shows that Ec and Ev are not flat but have curves.

 

•This indicates the presence of a voltage difference and is called built-in voltage.

 

•It always exists at the boundary and is determined by Na (Acceptor concentration) and Nd (Donor concentration).

 

•Figure 2. Built-In Voltage

 

 

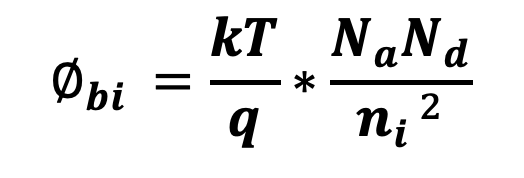
4.1.3 Poisson Equation

 

•Just as differentiating distance with respect to time gives velocity, and differentiating velocity with respect to time

gives acceleration, voltage behaves similarly.

 

•Differentiating voltage gives an electric field, and differentiating the electric field once more gives charge.

 

•This is called Poisson's Equation.

 

•The method involves finding the charge by subtracting the electric field entering and leaving a unit volume.

 

•Poisson's Equation is useful for finding the potential distribution when the charge density is given.

 

 

그림 3. Poisson's Equation

 

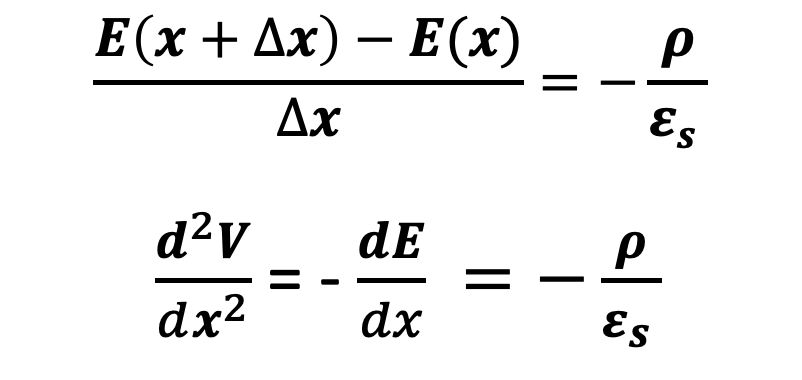
Reference 

-. Chenming Calvin Hu, Modern Semiconductor Devices for Integrated Circuits, PEARSON(2013)